Kominers’s Conundrums: The Great TV Compromise of 2021
Kominers’s Conundrums: The Great TV Compromise of 2021
(Bloomberg Opinion) -- We’re all feeling a bit cooped up these days, and stresses among some roommates are running high. But even in the most heated controversy, there is often more common ground than we might think.
This week’s Conundrum, co-authored with physicist Barbara Yew, is an example to prove the point. We’ve imagined a shared dwelling that — horrors! — has only one television. Some of the roommates want to watch the NFL playoffs, but others insist on watching reruns of the New Year’s Eve celebration for the umpteenth time.
The argument spins out of control, and eventually each side is accusing the other of hating everything they enjoy.
And what do they enjoy? The corresponding phrases in the left and right columns below might seem totally different at first, but you’ll find a surprising amount of commonality between them if you look closely.
If you figure out what’s going on, you should be able to resolve the differences between these housemates and determine the answer to this question: What do football fans and New Year’s enthusiasts have in common? That key to household harmony is this week’s answer.
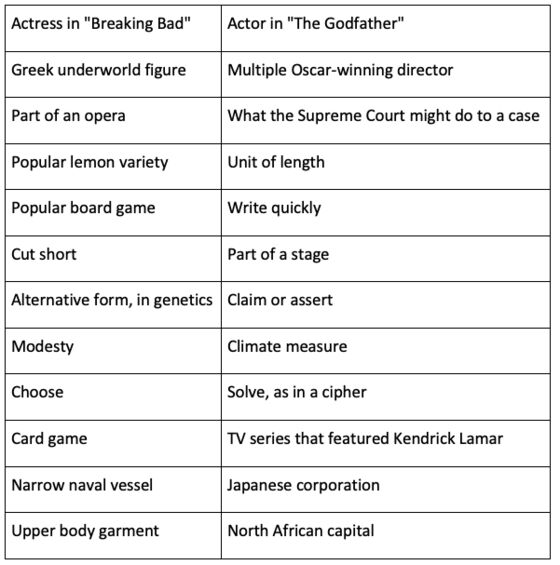
If you manage to figure out what ties the two groups together — or if you even make partial progress — please let us know at skpuzzles@bloomberg.net before midnight New York time on Thursday, January 21.
If you get stuck, there’ll be hints announced on Twitter and in Bloomberg Opinion Today. To be counted in the solver list, please include your name with your answer.
Programming note: Next weekend is one of the biggest puzzle events of the year — the MIT Mystery Hunt — which means that many of our solvers (as well as our host) will be otherwise engaged. Thus, the next Conundrums will run on January 24.
Previously in Kominers’s Conundrums …
To kick off the new year, we asked for your most inventive and ingenious constructions of the number 2,021. Any mathematical operation was fair game, but solvers were constrained to using factors of 36 (1, 2, 3, 4, 6, 9, 12, 18, and 36 — possibly using a given factor multiple times).
We sought submissions in two categories: Challenge, with the goal of getting 2,021 using as few factors of 36 as possible, and Creative, judged on simplicity and mathematical elegance.
The judging process for the Creative Category is still ongoing; we’ll be announcing those results in the next Conundrums column. In the meantime, here are the results for the Challenge Category:
Many solvers found five-factor solutions, such as 2^(9 + 2) – (3 * 9) = 2,048 – 27 = 2,021 and (6 * 36 + 9) * 9 – 4 = 2,025 – 4 = 2,021. Scott Wu managed to bring the latter of these constructions down to four factors, in the form (36 + 9)^2 – 4 = 2,025 – 4 = 2,021. Ross Rheingans-Yoo took that idea even further, observing that 45 is the ninth triangular number, constructed by creating successively larger stacks of dots in the shape of a triangle. That means you can use just three factors to get (Triangular(9))^2 – 4 = 2,025 – 4 = 2,021 (where here, “Triangular(N)” of course gives the N-th triangular number).
Three factors was the best any of the competition judges had managed to achieve. (In particular, Noam Elkies found that 2,021 = C(4!,3) – 3, where 4! = 24 is four factorial, and C(4!,3) = 2,024 then denotes the number of ways of choosing three items out of a set of 24.)
But our readers managed to do better!
To get to a solution using only two factors, Zoz observed that the 18th prime is 61, and converting 61 into base 3 yields 2,021 (albeit interpreted in base 3 rather than the usual base 10): Base3(NthPrime(18)) = 2,021.
And meanwhile Tanya Otsetarova achieved a particularly lucky two-factor solution: 2,021 = 43 * 47 = LuckyPrime(6) * LucasPrime(6), where “LuckyPrime(N)” and “LucasPrime(N)” respectively give the N-th prime elements of the Lucky and Lucas number sequences. (Lucky numbers are generated through a curious numerical elimination process; I described them a few years ago as we bade goodbye to 2018. The Lucas numbers, meanwhile, are closely linked to Fibonacci.)
So is two factors as far as you can go? Not by half!
Michael Thaler rose to the Challenge, winning the category with a one-factor construction. 2021 = SeeSay(3), where here “SeeSay(N)” refers to the “see it, say it” sequence that is constructed by starting at 1 and reading successive numbers in the sequence aloud by digit, including leading zeros.
It might seem a bit of an end run around the problem to find a sequence of which 2021 is the third element. But in fact, it’s actually quite remarkable that 2021 appears so early in a nontrivial integer sequence — much less one that’s so intuitive. And that sequence grows very quickly, so we won’t be see another year that overlaps with it for a very, very long time (SeeSay(4) = 0012101211).
Thanks to all those who submitted solutions, and congratulations to our most successful Challengers. We’ll be back with the Creative Category results — as well as the solution to this week’s Conundrum — on January 24.
The Bonus Round
In-browser quantum mechanics; a new weekly cryptic crossword at The Browser. Thirty years of “Jeopardy!” video games; Alex Trebek's banter; and a final “Jeopardy!” farewell tribute. 2021: a space Odyssey? Virtual animal encounters; the platypus genome; please help choose the Massachusetts state dinosaur (all three hat tip: Ellen Dickstein Kominers). Lego cars that can climb obstacles; awesome math art; highest-res snowflake photos; plus “Escaping an Infinitude of Lions.” And inquiring minds want to know: Are Google’s recipe ratings any good?
Michaela Wilson also got down to four factors using concatenation —that is, the mathematical operation where you glue numbers together left-to-right: (18+2) || 2 || 1 = 20 || 2 || 1 = 2021, where “||” denotes the concatenation operation.
Indeed, 3^3 = 27; the 27th prime is 103; and the 103rd prime is 563.
This sequence is closely related to the autobiographical numbers, whose digits read out how many of each digit they contain; 2,020 (“two 0s, zero 1s, two 2s, and zero 3s”) is a rare exemplar.
Granted, this is technically the digit representation “2021” rather than the number “2,021” — but that’s certainly close enough for the purpose of our competition!
Lazar Ilic suggested it might be possible to find an alternative one-factor construction starting from 3 and iterating the floor, factorial, and square root functions in some order. He wasn’t able to give a construction — but we found one, which we’ll tell you about along with the Creative Category standouts.
This column does not necessarily reflect the opinion of the editorial board or Bloomberg LP and its owners.
Scott Duke Kominers is the MBA Class of 1960 Associate Professor of Business Administration at Harvard Business School, and a faculty affiliate of the Harvard Department of Economics. Previously, he was a junior fellow at the Harvard Society of Fellows and the inaugural research scholar at the Becker Friedman Institute for Research in Economics at the University of Chicago.
©2021 Bloomberg L.P.

